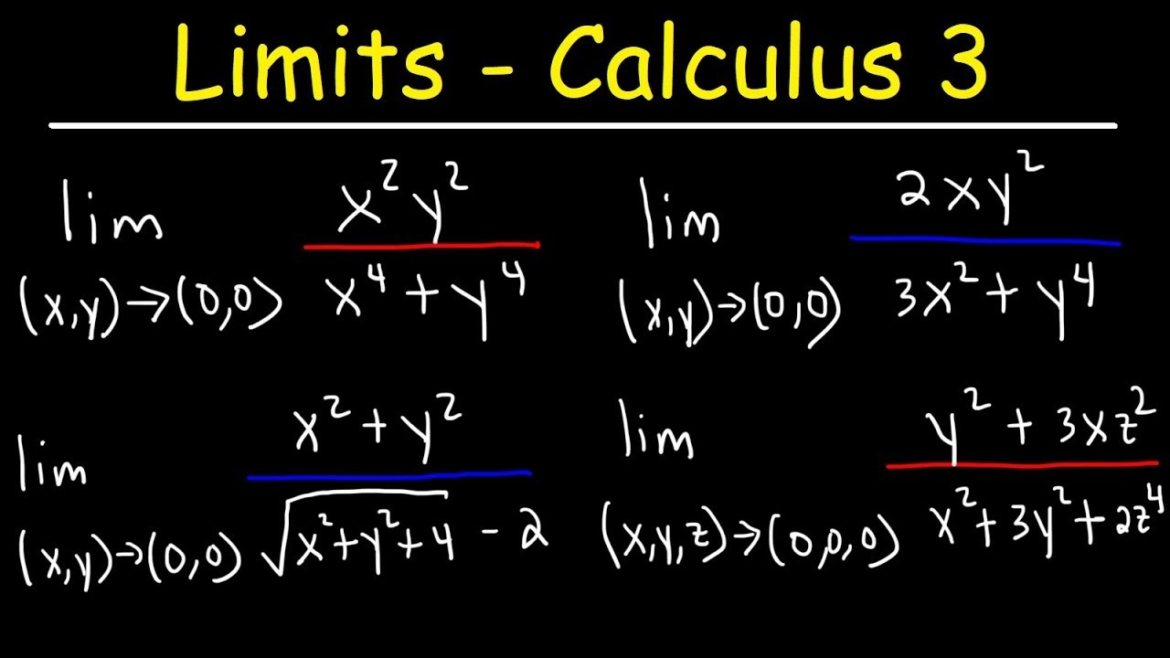
What is Limit Calculus and How to Calculate it? In calculus, the limit is one of the main types used to solve complex problems. It is widely used in continuity (as a condition, if it exists the function may be continuous), derivative (to find differential by first principle method), and integral (in definite integral).
The limit calculus finds the numerical value of the function at a typical point. In this article, we are going to describe the limit calculus along with examples and solutions.
Table of Contents
Limit Calculus – Definition
In calculus, a value that allows a function approach to some numeric value as the input of that function goes closer to a particular point is known as the limit. The value of the limit is obtained by placing the value of the given point in the place of the independent variable.
What is Limit Calculus and How to Calculate it? Limit calculus is not applied to the constant function as there must be a corresponding variable in that function in which the particular value is to be substituted.
Formula of Limit Calculus
Here is the general expression of finding the numeric value of the function.
Limt→c p(t) = M
- “t” is the independent variable of the function.
- p(t) is the given function.
- “c” is the particular point that w approaches.
- The final result after substituting the limit value is “M”
Also read :
Top 6 Best Platforms to Take Free Online Courses
How to Transfer Money from SweatCoin to Bank Accounts?
Laws of Limit Calculus
Here are a few well-known rules of limit calculus.
1. Sum Law:
When the function is given along with a plus sign among them then the notation of limit is applied to each function separately by using the sum law. According to this law, the general expression is:
Limt→c [p(t) + q(t)] = Limt→c [p(t)] + Limt→c [q(t)]
2. Power Law:
The power law of limit calculus is used when the function is given with an exponent. According to this law, the general formula is:
Limt→c [pn(t)] = Limt→c [p(t)]n
3. Constant Law:
The limit is not applied to the constant function and the function remains unchanged after applying the limit value because there is no independent variable available in the constant function. According to this law, the general formula is:
Limt→c k= k,
where k is any constant
4. Product Law:
When the function is given along with a product sign among them then the notation of limit is applied to each function separately by using the product law. According to this law, the general expression is:
Limt→c [p(t) * q(t)] = Limt→c [p(t)] * Limt→c [q(t)]
5. Constant function Law:
The constant function law of limit calculus is used to take the constant coefficients of the function outside the limit notation. According to this law, the general expression is:
Limt→c [k * p(t)] = k Limt→c [p(t)]
6. Difference Law:
When the function is given along with a minus sign among them then the notation of limit is applied to each function separately by using the difference law. According to this law, the general expression is:
Limt→c [p(t) – q(t)] = Limt→c [p(t)] – Limt→c [q(t)]
7. Quotient Law:
When the function is given along with a division sign among them then the notation of limit is applied to each function separately by using the quotient law. According to this law, the general expression is:
Limt→c [p(t) / q(t)] = Limt→c [p(t)] / Limt→c [q(t)]
8. L’hopital’s Law:
L’hopital’s law of the limit calculus is used when the function gives 0/0 or inf/inf form while solving the limit problems. According to this law, the derivative of the numerator and denominator must be taken and apply the limit value again.
If the function again gives a 0/0 form, then take the derivative again. According to this law, the general expression is:
Limt→c [p(t) / q(t)] = Limt→c [d/dt p(t) / d/dt q(t)]
How to solve the problems of limits?
The problems of the limit can be solved by using the below two ways.
- By using a limit solver
- By using a manual method
Let us describe both ways.
By using a limit solver
What is Limit Calculus and How to Calculate it? There are hundreds of online calculators are available to help students for getting step-by-step solutions to their complex problems. The problems of limit calculus can be solved easily by using a limit calculator with steps.
How to use this calculator?
Follow the below steps.
- Enter the function into the required input field.
- Select the corresponding variable “x” is selected by default.
- Select the type of limit.
- Enter the value of the limit.
- Press the calculate button.
- The solution with steps will come below the calculate button.
Manually
Below are a few limits calculus examples.
Example I
Calculate the limit of the given function if the particular value is 4
p(t) = 2t4 – 5t + cos(t) + 12wt5 + 15
Solution
Step I: Write the function along with the notation of the limit.
p(t) = 2t4 – 5t + cos(t) + 12wt5 + 15
c = 4
Limt→c [p(t)] = Limt→4 [2t4 – 5t + cos(t) + 12wt5 + 15]
Step II: Use the sum and difference laws of limit calculus and write the notation of limit to each function separately.
Limt→4 [2t4 – 5t + cos(t) + 12wt5 + 15] = Limt→4 [2t4] – Limt→4 [5t] + Limt→4 [cos(t)] + Limt→4 [12wt5] + Limt→4 [15]
Step III: Now take the constant coefficients outside the limit notation with the help of the constant function law.
= 2Limt→4 [t4] – 5Limt→4 [t] + Limt→4 [cos(t)] + 12w Limt→4 [t5] + Limt→4 [15]
Step IV: Now put t = 4 in the above expression to find the numerical value of the function by using the constant & power rules of limits.
= 2 [44] – 5 [4] + [cos(4)] + 12w [45] + [15]
= 2 [4 x 4 x 4 x 4] – 5 [4] + [cos(4)] + 12w [4 x 4 x 4 x 4 x 4] + [15]
= 2 [256] – 5 [4] + [cos(4)] + 12288w + 15
= 512 – 20 + cos(4) + 12288w + 15
= 492 + cos(4) + 12288w + 15
= 507 + cos(4) + 12288w
Example II
Calculate the limit of the given function if the particular value is 2
p(w) = 4w3 – 5w2 + 12w5 + 15w + 1
Solution
Step I: Write the function along with the notation of the limit.
p(w) = 4w3 – 5w2 + 12w5 + 15w + 1
c = 4
Limw→c [p(w)] = Limw→2 [4w3 – 5w2 + 12w5 + 15w + 1]
Step II: Use the sum and difference laws of limit calculus and write the notation of limit to each function separately.
Limw→2 [4w3 – 5w2 + 12w5 + 15w + 1] = Limw→2 [4w3] – Limw→2 [5w2] + Limw→2 [12w5] + Limw→2 [15w] + Limw→2 [1]
Step III: Now take the constant coefficients outside the limit notation with the help of the constant function law.
= 4Limw→2 [w3] – 5Limw→2 [w2] + 12Limw→2 [w5] + 15Limw→2 [w] + Limw→2 [1]
Step IV: Now put w = 2 in the above expression to find the numerical value of the function by using the constant & power rules of limits.
= 4 [23] – 5 [22] + 12 [25] + 15 [2] + [1]
= 4 [2 x 2 x 2] – 5 [2 x 2] + 12 [2 x 2 x 2 x 2 x 2] + 15 [2] + [1]
= 4 [8] – 5 [4] + 12 [32] + 15 [2] + [1]
= 32 – 20 + 384 + 30 + 1
= 12 + 384 + 30 + 1
= 396 + 30 + 1
= 427
Final Words
Now you can grab all the basics of limit calculus What is Limit Calculus and How to Calculate it? and find its problems easily. In this post, we have given a calculator and manual method along with examples to get the result of limit calculus easily.